
Total time = time upstream + time downstream = 3 hours

(to travel 8 km at 4 km/h takes 8/4 = 2 hours, right?) We can turn those speeds into times using: when going downstream, v = x+2 (its speed is increased by 2 km/h).when going upstream, v = x−2 (its speed is reduced by 2 km/h).Let v = the speed relative to the land (km/h)īecause the river flows downstream at 2 km/h:.Let x = the boat's speed in the water (km/h).There are two speeds to think about: the speed the boat makes in the water, and the speed relative to the land: What is the boat's speed and how long was the upstream journey? The negative value of x make no sense, so the answer is:Įxample: River Cruise A 3 hour river cruise goes 15 km upstream and then back again. The desired area of 28 is shown as a horizontal line. Let us solve this one by Completing the Square. How many you sell depends on price, so use "P" for Price as the variable what is the best price? And how many should you make? at $350, you won't sell any bikes at all.

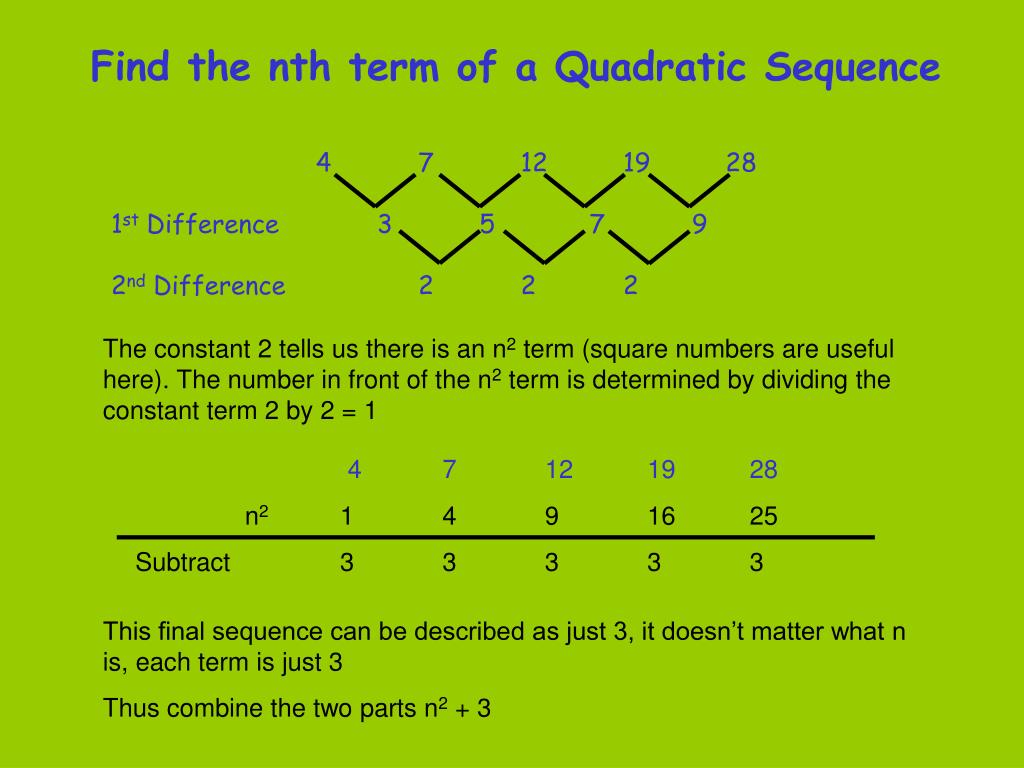
The method is explained in Graphing Quadratic Equations, and has two steps:įind where (along the horizontal axis) the top occurs using −b/2a: Note: You can find exactly where the top point is! The factors of −15 are: −15, −5, −3, −1, 1, 3, 5, 15īy trying a few combinations we find that −15 and 1 work Multiply to give a×c, and add to give b" method in Factoring Quadratics: There are many ways to solve it, here we will factor it using the "Find two numbers that It looks even better when we multiply all terms by −1: (Note for the enthusiastic: the -5t 2 is simplified from -(½)at 2 with a=9.8 m/s 2)Īdd them up and the height h at any time t is:Īnd the ball will hit the ground when the height is zero: Gravity pulls it down, changing its position by about 5 m per second squared: It travels upwards at 14 meters per second (14 m/s): (Note: t is time in seconds) The height starts at 3 m: You reason that the ball’s distance is a quadratic sequence.Ignoring air resistance, we can work out its height by adding up these three things:.You create the following table based on the photographs: Time (sec) You set up a camera to take a burst of photographs every second as a ball falls in front of a height chart. You are curious if there is a relationship between the number of feet a ball drops from a balcony \(144\) feet above ground. \) for some \(k\), Find \(k\) and \(p_n\).įor #28-30, refer to the experiment with gravity described below:


 0 kommentar(er)
0 kommentar(er)
